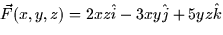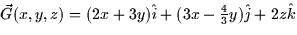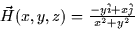Return to Other Sample Exams.

FOURTH HOUR MAKEUP EXAM
MATH 223
Instructor: Kris Green
May 5, 1998
10:00 - 10:50 am

1.

Consider the motion of a particle described by the parametric curve

$$x(t) = t + \cos t, \qquad y(t) = \sin t, \qquad 0 \le t \le 4\pi,$$

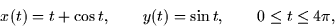
(a)

What is the particle's velocity?

(b)

At t = 0, the particle flies off along the tangent vector at constant velocity. Parameterize the resulting motion.

(c)

Another particle is traveling in a circle of radius 3, centered at (2,2) traversed counter-clockwise so that at t = 0 it is at (5,2). Give an implicit (ie. f(x,y)=0) form of this motion.

(d)

Will the motion of the first particle ever satisfy the relation that you found in (c)? If so, when?

(e)

What does your answer to (d) tell you about the motion of the two particles?

2.

(a)

Let x(t) and y(t) be given as shown below. Sketch a graph (including the starting point and direction of motion) for the motion in the xy plane.

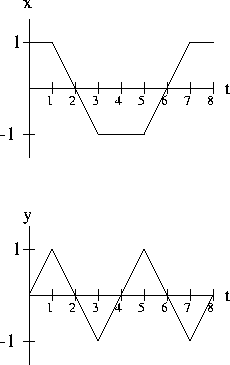
(b)

Using Green's Theorem, compute $\int_C [(2xy - 5y)\hat{i} + (x^2 - y^2)\hat{j}]\cdot d\vec{r}$ where C is the path in part (a). Note that you must modify Green's Theorem (slightly) to use it in this case.

3.

Given that

$$\mbox{curl} \vec{G} = 5\hat{k},$$

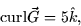
compute 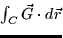$\int_C \vec{G} \cdot d\vec{r}$ where C is the triangle in the plane z = (17/10) - (3/5)x - (1/10)y with vertices at (1,1,1), (-1,3,2), and (-2,-1,3) traversed in this order. (Hint: Use Stokes' Theorem.)

4.

Which of the following vector fields are conservative? For those that are conservative, compute the scalar potential function. For those that are not, explain why they are not.

(a)

$\vec{F}(x,y,z) = 2xz \hat{i} - 3xy\hat{j} + 5yz\hat{k}$

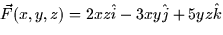
(b)

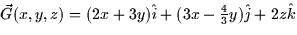
$\vec{G}(x,y,z) = (2x + 3y)\hat{i} + (3x - \frac{4}{3}y)\hat{j} + 2z\hat{k}$

(c)

$\vec{H}(x,y,z) = \frac{-y\hat{i} + x\hat{j}}{x^2 + y^2}$

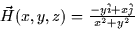
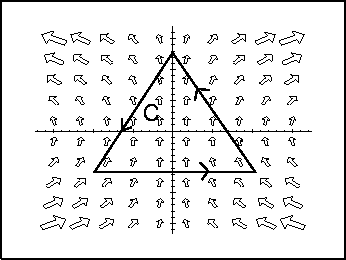
5.

For the illustrated paths and vector fields below, decide whether the line integral 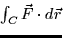$\int_C \vec{F} \cdot d\vec{r}$ is positive, negative, or zero.

(a)

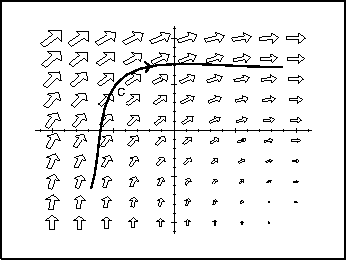
(b)

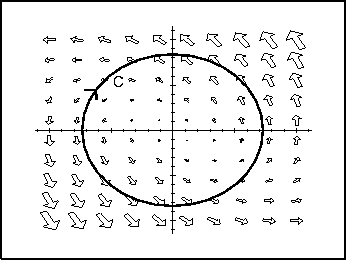
(c)

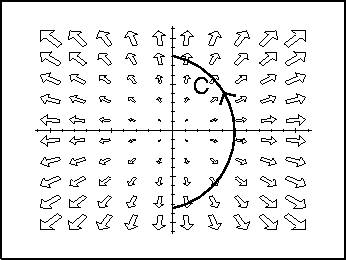
(d)