

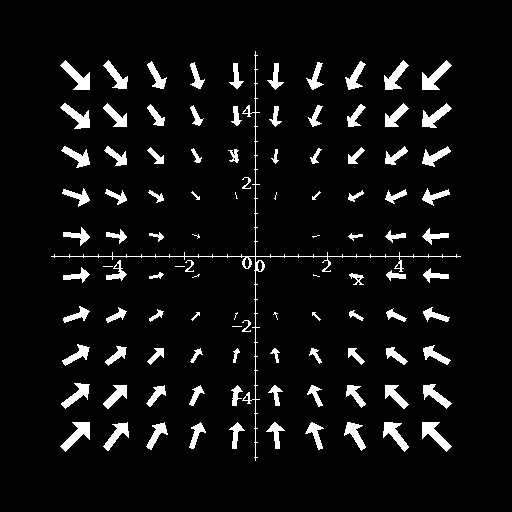
The divergence measures how much a vector field ``spreads out'' or diverges from a given point. For example, the figure on the left has positive divergence at P, since the vectors of the vector field are all spreading as they move away from P. The figure in the center has zero divergence everywhere since the vectors are not spreading out at all. This is easy to compute also, since the vector field is constant everywhere and the derivative of a constant is zero. The field on the right has negative divergence since the vectors are coming closer together instead of spreading out.


