223 Homework 4

Spring 1997

Due: Friday March 7, 1997

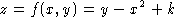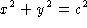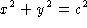Consider the function tex2html_wrap_inline18, constrained to tex2html_wrap_inline20. We can use Lagrange multipliers to find the maximum and minimum of f(x,y) constrained to tex2html_wrap_inline20.

  1. Draw the contour diagram of f and the constraint on the same coordinate axes.
  2. Based on your picture in part 1 how many solutions do you expect to have for the Lagrange multiplier problem?
  3. Label and classify each of these points on your diagram.
  4. Set up and solve the constrained optimization problem. Classify each of your solutions.