No Title

Math 223 Name
Fall 1997
Test 2

Directions: Please read each question carefully and show all your work in doing each question. No partial credit will be given if you do not show your work. If you have any questions about the test, please ask me. Your work should of course be your own. Good Luck!

1.

(15 points) Consider the function z = f(x,y) given by the table below. Find an approximate equation of the tangent plane to f at the point P = (1,0.5).

x $\setminus$ y	0	0.5	1	1.5	2
0	0	0.84	0.91	0.14	-0.76
0.5	0.25	1.09	1.16	0.39	-0.51
1	1	1.84	1.91	1.14	0.24
1.5	2.25	3.09	3.15	2.39	1.49
2	4	4.84	4.91	4.14	3.24

2.

(25 points) Consider the surface given by z = f(x,y) = y² e^x.

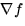(a): Find $\nabla f$ .
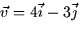(b): Find the directional derivative of f at the point P = (0,3) in the direction of $\vec{v} = 4 \vec{i} - 3 \vec{j}$ .
(c): Give a vector in the direction of the minimum directional derivative at the point
P = (0,3).
(d): Give a vector in the direction of zero directional derivative at the point P = (0,3).
(e): Consider the points P = (0,3) and Q = (1,4). Would you expect the contours of f to be closer together at P than they are at Q? Why or why not?

3.

(15 points)

=3ingraph7.ps

(a): Draw the coordinate axes for the xy-plane below and then draw a vector in the direction of the gradient at the point P = (1,0.5).
(b): Is the magnitude of the gradient larger at P = (1,0.5) or Q = (1,1.5)? Explain your reasoning.
(c): Would you expect 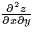$\frac{\partial^{2} z}{\partial x \partial y}$ to be positive or negative at Q = (1,0.5)? Explain your reasoning.

4.

(15 points) Consider the surface S given by 4xy²-2z = 1. Is the plane 16x + 16y - 2z = 33 tangent to S at the point $P = (1,2,\frac{15}{2})$ ? How do you know?

5.

(10 points) Consider a function z = f(x,y) where x = g(w,t) and y = h(w,t).

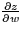(a): Write a general expression for $\frac{\partial z}{\partial w}$ .
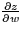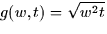(b): Use your expression above to find $\frac{\partial z}{\partial w}$ if f(x,y) = x + y², $g(w,t) = \sqrt{w^{2}t}$ , and
h(w,t) = 2t - w.

6.

(20 points) Compute all second order partial derivatives of the function
$z = f(x,y) = \sqrt{x-y^{2}}$ .

Vector Calculus
8/21/1998