The curl of a vector field measures the tendency for the vector field to swirl around. Imagine that the vector field represents the velocity vectors of water in a lake. If the vector field swirls around, then when we stick a paddle wheel into the water, it will tend to spin. The amount of the spin will depend on how we orient the paddle. Thus, we should expect the curl to be vector valued.
As other examples, consider the illustrations below. The field on the left,
called ![]() has curl with positive
has curl with positive ![]() component. To see this,
use the right hand rule. Place your right hand at P. Point your fingers
toward the tail of one of the vectors of
component. To see this,
use the right hand rule. Place your right hand at P. Point your fingers
toward the tail of one of the vectors of ![]() . Now curl your fingers
around in the direction of the tip of the vector. Stick your thumb out.
It points toward the +z axis, so the curl should have positive
. Now curl your fingers
around in the direction of the tip of the vector. Stick your thumb out.
It points toward the +z axis, so the curl should have positive ![]() component.
component.


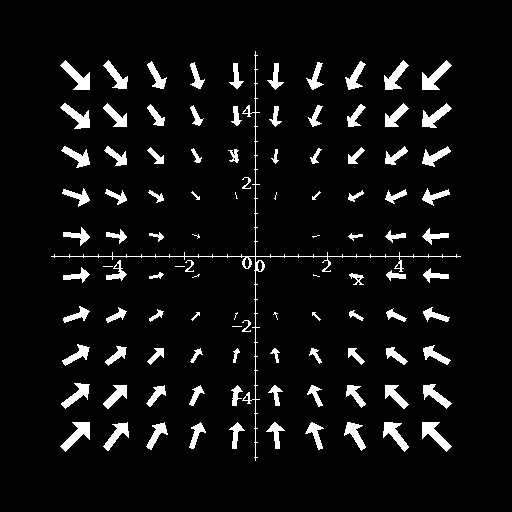
The second vector field has no swirling tendency at all (from visual
inspection) so we would expect ![]() . The
third vector field doesn't look like it swirls either, so it also has zero
curl.
. The
third vector field doesn't look like it swirls either, so it also has zero
curl.