Double integrals

Suppose we have a change of coordinates given by

$$x = x(u,v), \qquad y = y(u,v).$$

What is the area element, dA, in the uv coordinate system?

Note that a region in uv-space that is rectangular is described by constant functions of the uv coordinates. The same patch of the plane in xy coordinates, looks more like a parallelogram (if we make it small enough):

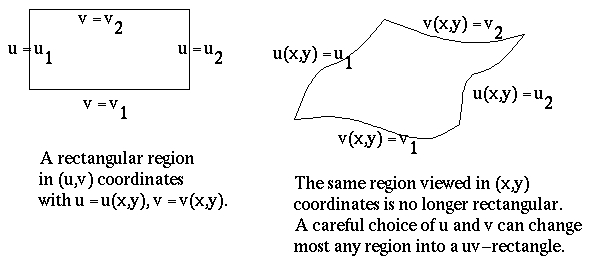
The area of the parallelogram in the Cartesian plane can be found by taking the magnitude of the cross product of two vectors that lie along non-parallel edges of the figure. Thus,

$$\Delta A \approx \vert\vert \vec{a} \times \vec{b} \vert\vert$$

where $\vec{a}$ and $\vec{b}$ are the displacement vectors along the sides of the parallelogram:

This gives us

We now define the Jacobian of the coordinate change, using determinants, to be $J = \frac{\partial (x,y)}{\partial (u,v)}$ given by

$$\frac{\partial (x,y)}{\partial (u,v)} = \frac{\partial x}{\p... ...\partial v} & \frac{\partial y}{\partial v}\end{array} \right].$$

The area element is then given by

$$\Delta A \approx \left\vert \frac{\partial (x,y)}{\partial (... ...t\vert \frac{\partial (x,y)}{\partial (u,v)} \right\vert du dv.$$

To convert an integral in xy to an integral in uv:

1.

substitute x = x(u,v), y = y(u,v) into the integrand to get this in terms of the new coordinates,

2.

find the boundaries of the region of integration in uv space, and

3.

let $dA = \left\vert \frac{\partial (x,y)}{\partial (u,v)} \right\vert du dv$.