


Next: More on breaking up
Up: The Order of Integration
Previous: The shape of a
Some functions are difficult (if not impossible) to integrate in a
particular order over some regions. For example, let's try to evaluate
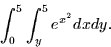
To integrate in the order given, we need to evaluate 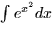 .This cannot be done. Ever. Thus, we have no choice but to attempt
reversing the order of integration.
.This cannot be done. Ever. Thus, we have no choice but to attempt
reversing the order of integration.
- 1.
- First, determine the boundaries of the region. As given in the
integral, we have
| Boundary |
Equation |
|---|
| left |
x = y |
| right |
x = 5 |
| bottom |
y = 0 |
| top |
y = 5 |
- 2.
- Next, we should draw the region.
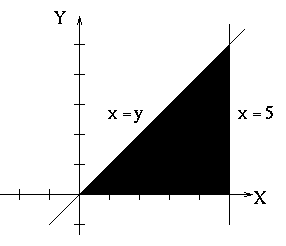
- 3.
- Find the bottom and top boundaries. The bottom is just y = 0. The
top is the line y = x.
- 4.
- Find the left and right boundaries. The left is just x = 0 and the
right is x = 5.
- 5.
- Now we set up the integral and evaluate it.
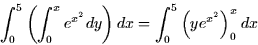
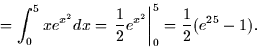
The clue that you need to switch the order of integration is the is you
switch it, you get an integrating factor which lets you evaluate the outer
integral by substitution.



Next: More on breaking up
Up: The Order of Integration
Previous: The shape of a
Vector Calculus
8/20/1998
![]()
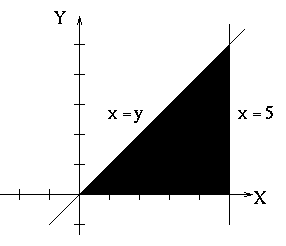
![]()
![]()